A first year-first semester course on group theory has its hilarious moments. Whereas they can relate the two other pure math courses (linear algebra and analysis) _somewhat_ to what they’ve learned before, with group theory they appear to enter an entirely new and strange world. So, it is best to give them concrete examples : symmetry groups of regular polygons and Platonic solids, the symmetric group etc. One of the lesser traditional examples I like to give is Nim addition and its relation to combinatorial games.
For their first test they had (among other things) to find a winning move for the position below in the Lenstra’s turtle turning game. At each move a player must put one turtle on its back and may also turn over any single turtle to the left of it. This second turtle, unlike the first, may be turned either onto its feet or onto its back. The player wins who turns the last turtle upside-down.
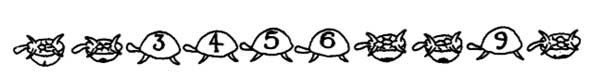
So, all they needed to see was that one turtle on its feet at place n is equivalent to a Nim-heap of height n and use the fact that all elements have order two to show that any zero-move in the sum game can indeed be played by using the second-turtle alternative. (( for the curious : the answer is turning both 9 and 4 on their back ))
A week later, one of the girls asked at the start of the lecture :
Are there real-life applications of group-theory? I mean, my father asked me what I was learning at school and I told him we were playing games turning turtles. I have to say that he was not impressed at all!.
She may have had an hidden agenda to slow me down because I spend an hour talking about a lot of things ranging from codes to cryptography and from representations to elementary particles…
For test three (on group-actions) I asked them to prove (among other things) Wilson’s theorem that is
$~(p-1)! \equiv -1~\text{mod}~p $
for any prime number $p $. The hint being : take the trivial action of $S_p $ on a one-element set and use the orbit theorem. (they know the number of elements in an $S_n $-conjugacy class)
Fingers crossed, hopefully daddy approved…
One Comment